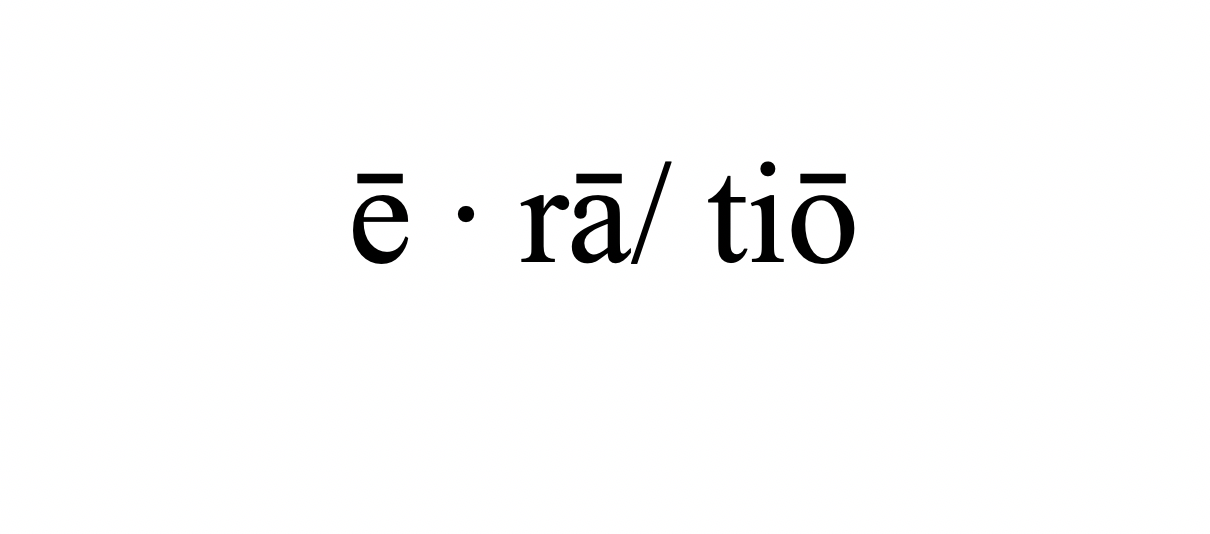A great quote by Douglas Hofstadter that I think is pertinent to the debate I’ve been having with Kaz Maslanka and Bob Grumman over my use of analogy in my “mathematical poetry”:
“Analogical thought is dependent on high-level perception in a very direct way. When people make analogies, they are perceiving some aspects of the structures of two situations — the essences of those situations, in some sense — as identical. These structures, of course, are a product of the process of high-level perception.”
From the essay The Pedagogical and Epistemological Uses of Analogy in Poetry and Mathematics by Marcia Birken and Anne C. Coon (and which cites work by fellow mathematical poet JoAnne Growney).
For the debate, see the comments attached to my essays, “On Mathematical Poetry.”
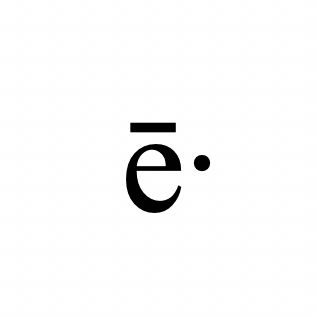
e·
Tuesday, June 29, 2010
Friday, June 25, 2010


Three works in sculpture by the American artist Joseph Keppler.
From the Seattle Group show Spirit Resonance at the Ouch My Eye Gallery, Seattle, WA.
e·
Thursday, June 24, 2010
Thanks go to JoAnne Growney for taking notice of my posts on mathematical poetry at her blog Intersections -- Poetry with Mathematics. I had the pleasure of meeting JoAnne at the Bowery Poetry Club at the opening of the Mathematical Graffiti wall a couple weeks ago on Friday, June 11. Thanks, JoAnne. What a pleasure meeting you!
“The processes of mathematics offer themselves to the Beckett protagonists as a bridge into number’s realm of the spectrally perfect, where enmired existence may be annihilated by essence utterly declared.”
— Hugh Kenner, Samuel Beckett A Critical Study
“The processes of mathematics offer themselves to the Beckett protagonists as a bridge into number’s realm of the spectrally perfect, where enmired existence may be annihilated by essence utterly declared.”
— Hugh Kenner, Samuel Beckett A Critical Study
Tuesday, June 22, 2010
The math-art graffiti wall at The Bowery Poetry Club is taking shape. (If you are reading this, you are invited to get down to the BPC and write some math-graffiti of your own!)
John Sims.
“Here is a poem I did with my NYU students in response to the Sol LeWitt / Adrian Piper show. Each student including myself wrote and recorded a small poem that was stitched together into a hyper poem. Visually each poem was mapped into a binary visualization of pi (see my pi quilt work — same idea). These crossword-looking forms were then mapped back into the original binary form (this gives a sense of self-similiarity as seen in fractals). Now, we created a model of this in animation space. The default flight path is associated with the sequence of pi in base two. As you travel over each section you will hear the voice of the student associated with that sub-poem. However the program allows for any path.” — John Sims
Wednesday, June 02, 2010
This Saturday, June 5, 2010, will be “Jack Foley Day” in Berkeley, California. On this day Jack Foley will receive a Lifetime Achievement Award from the Berkeley Poetry Festival .
Congratulations, Jack Foley.
Walking Across Brooklyn Bridge
by Jack Foley
As we walked across the Bridge,
I thought of Hart Crane
(poor suicidal poet)
& of Joseph Stella
whose paintings are like chords struck
on the harp strings
of this amazing edifice
Crane saw The Waste Land
as a condemnation
of modern industrial society
(“so negative”)
His answer was
this marvel
of spidery, delicate cables
which swoop us into skies
of unspeakable beauty
“sleepless as the river under thee”
(Eliot: “Fear death by water”)
Our guide, Gregory,
quietly told us stories,
raged against the sometimes reckless bikers,
& watched our wonder
as we walked
across this passageway
from busy life to busy life—
this “between”
suspended over water—
that took us
into the sky
& home to Brooklyn
Congratulations, Jack Foley.
Walking Across Brooklyn Bridge
by Jack Foley
As we walked across the Bridge,
I thought of Hart Crane
(poor suicidal poet)
& of Joseph Stella
whose paintings are like chords struck
on the harp strings
of this amazing edifice
Crane saw The Waste Land
as a condemnation
of modern industrial society
(“so negative”)
His answer was
this marvel
of spidery, delicate cables
which swoop us into skies
of unspeakable beauty
“sleepless as the river under thee”
(Eliot: “Fear death by water”)
Our guide, Gregory,
quietly told us stories,
raged against the sometimes reckless bikers,
& watched our wonder
as we walked
across this passageway
from busy life to busy life—
this “between”
suspended over water—
that took us
into the sky
& home to Brooklyn
With Jack Foley in NYC, 2005.
Subscribe to:
Posts (Atom)